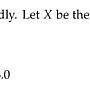: 想問雙重期望值怎麼得出8 有點不太懂
: 骰出5可能要一萬次 不知道怎麼算平均所需要的次數
8 的來源:
> So given that a 5 is not rolled on the first roll, an average of 7
> additional rolls will be needed
在第一骰不是 5 的狀況下, 還需要平均 7 骰 => 所以總計是 8
那 7 怎麼來的?
> -- the wasted second roll and the usual average of 6 rolls to get the
> number you want.
肯定是 6 的第二骰 (Y=2) 跟後面平常的幾何分佈平均要 6 骰, 總計 7 骰
至於雙重期望值就只是單純下面的式子, 它是這樣來的:
令 Z 表示第一骰是否為 5, 則下面式子在算的是:
E[X|Y=2] = E[ E[X|Y=2, Z] ]
= Pr(Z 真) * E[X|Y=2, Z 真] + Pr(Z 假) * E[X|Y=2, Z 假]
\ / \ / \ / \ /
0.2 1 0.8 上面討論的 8
: https://i.imgur.com/I7PNbuJ.png
: 第二個是不懂為什麼這樣可以代表p（x大於10)
: 感謝大大
它上一行的數學式推導出了 Pr(X = k) = Pr(X = 20-k)
也就是 X=0 的機率等於 X=20 的機率, X=1 的機率等於 X=19 的機率, 等等
所以 Pr(X<10) = Pr(X=0) + Pr(X=1) + ... + Pr(X=9)
= Pr(X=20) + Pr(X=19) + ... + Pr(X=11) = Pr(X>10)
接下來的推論就是上面涵蓋了所有 21 種狀況中的 20 種, 只除了 Pr(X=10)
因此 1-Pr(X=10) 即是上述兩半邊的和, 那兩半邊又相等所以除以 2 即可求得
--
'You've sort of made up for it tonight,' said Harry. 'Getting the
sword. Finishing the Horcrux. Saving my life.'
'That makes me sound a lot cooler then I was,' Ron mumbled.
'Stuff like that always sounds cooler then it really was,' said
Harry. 'I've been trying to tell you that for years.'
-- Harry Potter and the Deathly Hollows, P.308
--
※ 發信站: 批踢踢實業坊(ptt.cc), 來自: 123.194.181.180 (臺灣)
※ 文章網址: https://www.ptt.cc/bbs/Math/M.1696888303.A.EFA.html
※ 編輯: LPH66 (123.194.181.180 臺灣), 10/10/2023 05:58:29
→
10/10 08:46,
6月前
, 1F
10/10 08:46, 1F
→
10/10 08:48,
6月前
, 2F
10/10 08:48, 2F
→
10/10 08:48,
6月前
, 3F
10/10 08:48, 3F
→
10/10 08:48,
6月前
, 4F
10/10 08:48, 4F
推
10/10 08:51,
6月前
, 5F
10/10 08:51, 5F
→
10/10 08:55,
6月前
, 6F
10/10 08:55, 6F
→
10/10 08:55,
6月前
, 7F
10/10 08:55, 7F
討論串 (同標題文章)
